Marx (1894) introduced the concept of "production price" as a result of the full mobility of capital between different sectors of production toward the equalisation of its gain rates. Nevertheless, he doesn't explain this process of equalisation between heterogeneous sectors, and its Law of Decreasing Tendency of the Gain Rate supposes an unjustified equalisation of the plusvalue rates (quotient between the gain and the capital invested in work power).

After, Sweezy (1972) and Salama-Valier (1973) intended to explain the process, but their explanations weren't satisfactory for treating with static situations in equilibrium, when the real evolution is produced from situations of non-equilibrium.

Pla-Lopez (1986) presented a model of System Dynamics of the equalisation of the Gain Rate, which explained the possibility of evolution toward a greater proportion of inversion in production means, and concluded that the Marx's Law of Decreasing Tendency of the Gain Rate was wrong.

Now, we are going to complete the model by introducing the adaptation of the Demand to the Incomes, which we name Solvent Demand, and studying by computer simulation the results of the model in the evolution of the Main Gain Rate.
 

We work with classical curves of Supply and Demand (see for example Lipsey (1967)), with its "elasticity" as exponent. These curves ideally would determine the Supply and Demand in function of the prices,
        Of = k_Opr^Eo
        Dem = k_D pr^Ed
Of course, usually the elasticity of the Supply will be positive, Eo>0, and the elasticity of the Demand will be negative, Ed<0. But the condition of equilibrium is that the elasticity of the Demand would be greater in absolute value than the elasticity of the Supply, |Ed|>|Eo|.
In order to simulate the evolution of the Supply, we suppose that the Ideal Supply is determined by the real price, and the real Supply Of tends lineally toward the Ideal Supply Of with a delay t1, so that
(1)    Of_i = k_Oⁱ pr_i^Eo
(2)    Of_i(t+1) = Of_i(t) + (Of^I_i(t) - Of_i(t))/t₁
for every sector "i". Of course, if the delay t1 is greater, the evolution of the Supply to its Ideal value will be slower, and if t₁=1 then the Supply equals its Ideal value in one step.

Also, in order to simulate the evolution of the Demand to its Ideal value with equalisation of Supply and Demand, we will suppose that the Ideal Demand is equal to the real Supply, the Ideal Price is determined by the Ideal Demand according to the relation (1'), and the real Price tends lineally toward the Ideal Price with a delay t₂, that is to say
(3)    Dem^I_i = Of_i
(4)    pr^I_i = (Dem^I_i / k_Dⁱ)^1/Ed
(5)    pr_i(t+1) = pr_i(t) + (pr^I_i(t) - pr_i(t))/t₂
(6)    Dem_i = k_Dⁱpr ^Ed

If the condition of equilibrium |Ed|>|Eo| is fulfilled, then this System will evolve toward an situation of equilibrium in which
Dem = Dem ^I = Of = Of ^I = (k_D^Eo k_O^-Ed)^1/(Eo-Ed)
and
pr = pr^I = (k_D/k_O)^1/(Eo-Ed).
 

We will suppose that to produce a unity of the product of the sector "i" we need a^ij unities of the sector "j", so that its unitary cost is
(7)    kⁱ = Σ_j a^ij pr_j
for every sector "i". We consider work power as a sector, so that wages are included in this unitary cost.

The total cost in a sector "i" will be kⁱ Of_i , but its total incomes will be pr_i mⁱ, where mⁱ will be the minimum between its Supply and its Demand,
(8)    mⁱ = min ( Of_i, Dem_i )
Therefore, the gain rate in this sector will be gⁱ = (pr_i mⁱ - kⁱ Of_i)/ kⁱ Of_i = pr_i mⁱ / kⁱ Of_i - 1 .
Because mⁱ ≤ Of_i , gⁱ ≤ pr_i / kⁱ - 1 , and therefore, gⁱ > 0 only if Σ_j a^ij pr_j< pr_i . So, in order to get gⁱ>0 for every i, we can take a^ijso that ||a||<1, and therefore ||a pr||<||pr||. We will work with infinite norm, and so we will take a^ij so that Σ_j a^ij < 1 (the coefficients a^ij are always positive). This condition is not necessary, but if the condition å_j a^ij pr_j < pr_i  is fulfilled we can select the unity of each product in order to get it.

The Mean Gain Rate will be g_m = Σ_i gⁱ kⁱOf_i /Σ_j k^jOf_j , and therefore
(9)    g_m = Σ_i pr_i mⁱ/Σ_j k^j Of_j  - 1
If you launch the model with the relations (1) to (5) with the condition of equilibrium |Ed|>|Eo| and random coefficients a^ij so that Σ_j a^ij < 1 , the Mean Gain Rate increases until reaching the equilibrium between Supply of Demand (in fact, out of this equilibrium can inefficiently be mⁱ < Of_i , so that gⁱ < pr_i/ kⁱ  - 1 and g_m < Σ_i pr_i Of_i /Σ_j k^jOf_j -1 which is its value in this equilibrium). We can see an example in the Figure 1.

Figure 1 Evolution of Mean Gain Rate with a single adjustment of Supply and Demand

Nevertheless, in this simple equilibrium between Supply and Demand, with constant parameters kOi and kDi , the Gain Rates of the different sectors are not equalised. This equalisation requires a movement of capital between sectors which will produce a displacement of the curves of Supply, through a change of the parameters kOi , so that the prices of equilibrium between Supply and Demand will tend to the Production Prices ppi in each sector "i" so that its Gain Rate were equal to the Main Gain Rate,  gi = ppi / ki - 1 = gm , and therefore

(10)    pp_i = (1+g_m) kⁱ

In order to simulate the evolution of the parameters k_Oⁱ , we will suppose that they tend lineally with a delay t₃ to an ideal value k_O^I_i which corresponds to the Production Price pp_i with the present parameter k_Dⁱ of the curve of Demand, so that
(11)    k_O^I_i = k_Dⁱ /pp_i^Eo-Ed
(12)    k_Oⁱ (t+1) = k_Oⁱ (t) + (k_O^I_i(t) - k_Oⁱ (t))/t₃

Note that, in conditions of equilibrium, Σ_j a^ij pp_j = pp_i / (1+g_m), and therefore 1/(1+g_m) has to be a self-value of the matrix a^ij , and the prices of production form its corresponding self-vector. Therefore, the possible values of Mean Gain Rate in equilibrium, and also the relative proportion of the prices in equilibrium, depend only on the intersectorial coefficients a^ij (Pla-Lopez 1986).

The relative proportions of the parameters in equilibrium of the curves of Supply, k_Oⁱ , will also depend on the parameters of the curves of Demand, k_Dⁱ. Of course, according to the relations (11), if the prices in equilibrium change in a proportion λ from different initial conditions, these parameters in equilibrium will change in a proportion λ^Ed-Eo.
 
 

Nevertheless, a Demand is only sustainable if it is Solvent, that is to say, it can be paid for the corresponding Incomes. In order to study the adaptation to the Solvent Demand, we will consider only three sectors:
Sector 0: production of means of production.
Sector 1: production of goods for workers.
Sector 2: production of goods for owners of means of production.
In order to simplify the model, we will suppose that there is a full division between workers and owners of means of production, and between sector 1 and sector 2.

In the relations (6), the prices of sector 2 don't contribute to the unitary cost of any product, and therefore aⁱ²=0 for every sector "i". By the way, the prices of sector 1 contribute to the unitary cost through the wages (see Sraffa 1960). So, the total incomes of the workers (its wages) will be equal to the total cost from the sector 1, Σ_i Ofi aⁱ¹ pr₁ . Therefore, the Solvent Demand of the sector 1 will be Dem^S_i = Σ_i Of_i aⁱ¹ pr₁/ pr₁ = Σ_i Of_i aⁱ¹ and according to the relation Dem = k_D pr^Ed  its parameter of Solvent Demand will be
(13)    k_SD¹ = Dem^S₁/pr₁^Ed = Σ_i Of_i aⁱ¹ / pr₁^Ed
and we will suppose that the parameter k_D¹ tends lineally to this parameter of Solvent Demand with a delay t₄ ,
(14)    k_D¹(t+1) = k_D¹(t) + (k_SD¹(t) - k_D¹(t))/t₄

On the other hand, the total incomes of the owners of means of production will be its total gain,
Σ_i pr_i mⁱ - Σ_i kⁱ Of_i. Therefore, the Solvent Demand of the sector 2 will be
Dem^S₂ = (Σ_i pr_i mⁱ- Σ_i kⁱ Of_i )/ pr₂ , and the corresponding parameter of Solvent Demand will be
(15)    k_SD² = Dem^S₂ / pr₂^Eo = (Σ_i pr_i mⁱ - Σ_i kⁱ Of_i)/ pr₂^Eo+1
We will also suppose that the parameter k_O² tends lineally to this parameter of Solvent Demand with a delay t₄ ,
(16)    k_D²(t+1) = k_D²(t) + (k_SD²(t) - k_D²(t))/t₄

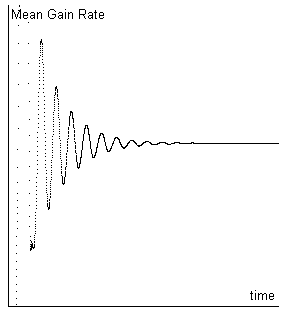Figure 2 Evolution of Mean Gain Rate with static technology

The interaction between the adjustment of Supply and Demand, the equalisation of the Gain Rates and the adjustment to the Solvent Demand not always tends toward equilibrium. But if so, the Mean Gain Rate oscillates toward its value of equilibrium, which only depend on the intersectorial coefficients which express the technological conditions. In Figure 2 you can see an example. On the other hand, in a solvent equilibrium  kD1 = kSD1 and kD2 = kSD2 and, because m=Of=Dem= kD prEd , if we name pij=ppi/ppj, according to the relations (13) and (15), then the parameters of the curves of Demand will have to fulfil the system of equations

(1-a¹¹) k_D¹ + a²¹ p₂₁^Ed k_D² = a⁰¹ p₀₁^Ed k_D⁰
(a¹⁰ p₀₂ + a¹¹ p₁₂ ) p₁₂^Ed k_D¹ + (a²⁰ p₀₂ + a²¹ p₁₂) k_D² = ( (1- a⁰⁰) p₀₂ - a⁰¹ p₁₂) p₀₂^Ed k_D⁰
Therefore, the relative proportions k'_D¹ = k_D¹/ k_D⁰ and k'_D² = k_D²/ k_D⁰ will have to fulfil the system of equations
(1-a¹¹) k'_D¹ + a²¹ p₂₁^Ed k'_D² = a⁰¹ p₀₁^Ed
(a¹⁰ p₀₂ + a¹¹ p₁₂ ) p₁₂^Ed k'_D¹ + (a²⁰ p₀₂ + a²¹ p₁₂) k'_D² = ( (1- a⁰⁰) p₀₂ - a⁰¹ p₁₂) p₀₂^Ed
And, provided that this system of equations were determined, because p_ij depend only on the intersectorial coefficients a^ij , these relative proportions of the parameters of the curves of Demand in equilibrium will only depend on these intersectorial coefficients. Nevertheless, the process of evolution toward this equilibrium is only possible if both are positive.
 

Remember, but, that the unitary cost of a product is kⁱ = aⁱ⁰ pr₀ + aⁱ¹ pr₁, where aⁱ⁰ expresses the contribution of the means of production, and aⁱ¹ expresses the contribution of the work power through the wages (equal to aⁱ¹ pr₁). Therefore, these coefficients also express the social relations between workers and owners of means of production, and the unionist struggle can carry to increase the wages, and so it could carry to increase the coefficients ai1. Nevertheless, we can plausibly suppose that the wages increase lesser than the productivity, and so that the coefficients ai1 do not increase, but decrease through technological progress, by means of saving in work power.

The Model uses Ed=-.5 and Eo=.3 (but these values can be easily changed), and demands to fix the values of the delays (we have used t₁=t₂=t₄=10 and t₃=t₅=20). And, in order to simulate the process without more presuppositions, the Model can fix randomly the initial values of intersectorial coefficients, prices, amount of Supply and parameters of the curves of Supply and Demand. Then, the dynamical relations of the Model can change these values, eventually toward some value of equilibrium of the Mean Gain Rate.

Nevertheless, the technological progress, through a random decreasing of the intersectorial coefficients, can break this equilibrium, and begin an evolution toward a new equilibrium. You can see a typical process in Figure 3: through this technological progress, the Mean Gain Rate increases over its previous value of equilibrium.

You can find the source code of our Model in http://www.uv.es/~pla/models/modpp.c , and a DOS executable program in http://www.uv.es/~pla/models/modpp.exe . This is an ANSI C program with only text output, but, of course, you can adapt the source code to your compiler in order to get a graphical output, like we have done.
 

Through our computer simulation, we have tested and ratified our previous conclusion (Pla-Lopez 1986) about the increasing of the Mean Gain Rate through technological progress. Moreover, its oscillations can explain some economic crisis.

Nevertheless, our Model explores a theoretical situation, without monopolies nor ecological bounds of the economic growth. These questions remain open to later studies, applications and developments of our Model.
 

Forrester, J:W. (1968), "Principles of systems", Wright-Allen Press, Cambridge.

Lipsey, R.G. (1967), "Introducción a la economía positiva", Vicens-Vives, Barcelona.

Marx, K. (1894), "El Capital", vol.III, Fondo de Cultura Económica, Mexico, 1959.

Pla-Lopez, R. (1986), "Study by System Dynamics of the Problem of the Equalization of the Gain Rate" in "System Dynamics: on the move", proceedings of the 1986 International Conference of the System Dynamics Society, Sevilla.

Salama, P. and Valier, J. (1973), "Une introduction a l'économie politique", Maspero, Paris.

Sraffa, P. (1960), "Production of Commodities by means of Commodities", Cambridge University Press, Cambridge.

Sweezy, P.M. (1972), "Teoría del desarrollo capitalista", Fondo de Cultura Económica, Mexico.