Two Sample T-Tests

Copyright © 1997 by Forrest W. Young.

  • Review of One-Sample Tests

    • Chapter 8 and Chapter 9 presented the statistical procedures that permit researchers to use a single sample mean to test hypotheses about a population. These statistical procedures were based on a few basic notions, which are summarized as follows:

    1. A sample mean is expected to more or less approximate its population mean. This permits us to use the sample mean to test a hypothesis about the population mean.

    2. The standard error provides a measure of how well a sample mean approximates the population mean. If we know the population standard deviation or variance, the standard error formula is:

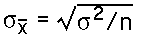
      If we don't know the population standard deviation or variance we use the sample's standard deviation or variance to obtain an estimate of the standard error. The formula for the estimate of the standard error is: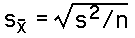

    3. To quantify our inferences about the population, we compare the obtained sample mean with the hypothesized population mean. If we know the population standard deviation or variance we compute a z-test statistic. The formula is:

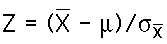
      If we don't know the population standard deviation or variance we compute a t-test statistics. The formula is: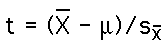

  • Review of The Logic of Hypothesis Testing

    • The overall process of hypothesis testing is the same whether or not we know the population standard deviation/variance: We have the same four steps.

    The details of some of the steps differ: The method of determining the critical region depends which one-sample test we are using, and, of course, we way we calculate the (estimate of the) standard error differs for T and Z.

    1. State the Hypotheses:
      This step is the same for both one-sample tests. We actually state two hypotheses:
      • Ho: The null hypothesis This states there is no effect (two-tail), or that the effect is not in the direction we anticipate (one-tail).
      • H1: The alternative hypothesis. This hypothesis states that there is an effect (two-tail), or that the effect is in an anticipated direction (one-tail).

    2. (Classical Approach): Set the decision criteria.
      • Specify the significance level.
        This is the same for both one-sample tests. Often times we state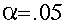
      • Determine the critical value. The details depend on the test:

        Z-Test: We use the alpha-level to find the critical Z value in the Z table.

        T-Test: We use the alpha-level and the degrees of freedom to find the critical T value in the T table.

        Notice that there is a new complication in using T: There isn't just one T-distribution that we use to determine the critical value of T. There is a whole family of distributions. The distribution depends on the "degrees of freedom".

        For the one-sample T-test, the degrees of freedom is simply equal to one less than the sample size. That is:

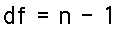
    3. Gather Data.
      This step is the same for both one-sample tests.

    4. Evaluate the Null Hypothesis .
      • Classical approach (used when doing problems by hand):
        1. Determine the (estimate of the) standard error of the mean.
        2. Calculate the test-statistic value (this is the "observed" test statistic value).
        3. Determine if the observed test-statistic value is in the critical region.
        4. Decision: If the observed test-statistic value is in the critical region, reject the null hypothesis Ho. If it isn't, do not reject the null hypothesis.
      • Modern Approach (used when using statistical software):
        1. Determine the observed test-statistic value and its exact significance level.
        2. Report the values and interpret their implications for the null hypothesis.