 |
 |
| Gregarious Impact | Individualist Impact |
ABSTRACT
They are many global models of evolution of humanity. Nevertheless, its real evolution has had a strong duality between orient and occident. In a last work(12) , the duality is reproduced by means of regional differences of some social behaviour, but it is somewhat a forced explanation.
In this work, we study a new approach to explain this duality, by combining changes in conditions of initialisation and the opposition gregariousness/individualism.
This model can help us to understand the implied processes in the humanity’s
evolution, and to explain the form in which the dualism limits the globalisation
or the globalisation can overcome the dualism, and if this eventual overcoming
is a consequence of the domination of a part or of the convergence of different
evolutionary lines.
Pla-López and Castellar-Busó have simulated:
The model is composed by means of the interaction of several mathematical systems, which express the relation between the variables involved:
The core of the model is the Learning System, through positive and negative reinforcement: the probability (P) of each social behaviour (U), in each individual subsystem (N)of a social population, increases when his goal is fulfilled and decreases when it is not fulfilled from this social behaviour. If no social behaviour available to an individual subsystem produces goal fulfilment, then this subsystem can be destroyed.
The fulfilment probability (PG) depends on its technical possibility (p ), which is weighted by a factor determined by the social organisation (1-s ).
This factor is generated by a Repression System: each social behaviour, according to its repressive capacity (S), its scope (F ) in each individual system and the impact (IMP) between individual subsystems, produces a decrease of this factor for the different social behaviours.
Thus, each social behaviour (U) represses the other social behaviours, by decreasing its goal fulfilment.
A Relay System produces a random (a ) substitution (g ) of an individual subsystem for a "child subsystem" with initial equiprobability (natal) of every available social behaviours. A "child subsystem" can also occupy (A) the niche of some destroyed system. Thus, relay causes the loss of the information accumulated in the substituted individual system.
Also, a Science System determines the probability of learning (PL) for each social behaviour in each subsystem: the probability of the experience of the other subsystems, which is weighted by factors of emission (EM), reception (RE) and impact (IMP), is added to its own experience. This system expresses the relations of intellectual communication between different individual subsystems.
A Historical System simulates historical evolution through the random (b ) increase (d ) of the dimension (m) of the state-variable in each subsystem, and therefore of the number of its available social behaviours. The probability of evolution is increased (b) by the existence of social behaviour, which were theoretically not available but are forced by system science from the experience of other subsystems. This system expresses technological progress and technological diffusion (we characterise a technologically higher society by a greater capacity of choice between different social behaviours).
An Adaptive System determines
A Resignation system expresses the influence of subjective factors through a tendency to a statistical normalisation of the reinforcement from satisfaction and dissatisfaction. We name this tendency "resignation", and express it by a time of delay (Tr) according to a model of systems dynamics: with a low delay Tr, the satisfaction will tend to be compared with its mean value, and the individuals subsystem tends to be resigned with global low values of satisfaction.
An Impact System express how the impact (IMP) on repression and intellectual communication depends on might m and distance (d)between subsystems.
A Natural System expresses a diversity of initial conditions (natal) of the individual systems. In its first version, this system is a rigid system, without neither feedback nor evolution.
Moreover, an Ecological System expresses the degradation of the environment as a consequence of the consumption in satisfaction and repression: the possibility of consumption decreases, so much in satisfaction like in repression, in order to recover the environment by means of the recycling.
The ecology is presented by means of a global variable (E) not negative. This variable will begin from an initial value (E0) sufficiently elevated like so that it were possible to be kept a consumption or in satisfaction or in repression, during all the evolution (E0= 2NP), as high as the whole of the active systems could sustain. Moreover, although the sum of this consumption during all the evolution can amount to the maximal level simultaneously, they won’t remain thus by cause of the degradation that the environment would suffer. Thus, this consumption in satisfaction and in repression contributes to the decrease of the resources, and so the variable E decreases and can reach the value zero (Ecological Hecatomb). Also, through processes of reutilization (r) and recycling (r ), it is possible the increase of this variable E, without overcoming its initial value E0 .
The difference between reutilization and recycling will be that the first didn’t include a cost which will characterise the second. This cost produces a decrease in the consumption in satisfaction, in repression or in both. Each individual system will tend to accept this cost by adapting its recycling to the ecological necessities with a delay Te .
Finally, a Delay System expresses the decrease of Adaptation Time Ta with might m and the increase of Resignation Time Tr with ferocity n , by means of the parameters Ka and Kr respectively. Moreover, Ecological Time Te increases with ferocity and decreases with might by means of a parameter Ke.
The mathematical relations are set out in Table 2.
2. DUALIZING MODEL
With the Adaptive, Historical, Geographical and Multidimensional Evolution with Resignation, we have studied the possible paths of global evolution through a Full Repressive Society to a Free Scientific Society. Nevertheless, in the real history of the humanity appears clear dualism between oriental and occidental evolutions.
In the last work(12), stimulating results have been obtained. Nevertheless, the approximation of regionalizing does not explain why the technical possibilities of satisfaction are different in different locations.
In the present work, we have changed the previous model by using a gregariousness/individualism approach and considering the influence of the evolution of the geographical natural conditions in order to modelling the outlined duality.
Now, we present the new relationships of the systems.
2.1. Repression System
Each social behaviour (U) represses the other social
behaviours, by decreasing its goal fulfilment. In the previous model, the
suffered social repression is
s(U,N)
= åU¹
V åMF(V,M)
sts(V,M) IMP(M,N)
where sts(U,N) is the repressive capacity of U
in a individual subsystem N .
F(U,N) =
P(U,N)2 /S
indicates the scope of U in the individual subsystem
N,
which a number S of active subsystems
IMP(M,N) is the impact of the system M
on
N,
IMP(M,N) = åU
P(U,M) imp(U,d)
where imp(U,d) is the impact of the behaviour
V
at the distance d (circular distance between the systems M,
N) .
We will express now the repression with the formula
s(U,N)=åU¹VåMF(V,M)
sts(V,M) imp(V,M,N)
where imp(V,M,N) is the impact of the behaviour
V
at the distance between the systems M, N .
2.2. Natural system
In the previous model(4,5,6,7,8,10,11), the Natural System appears isolated without input variables.
In this work, we will make that initial conditions (natal) depend on the ecological factor. For that reason, we have included a new factor, to which we call factor of the evolution (km). Then we have changed the definition of the Natural System:
A Natural System(15) determines the dynamic evolution of the initial conditions (natal) of each individual subsystem N, in case of ecological degradation, from an initial value (natal0) toward an ideal value (ntl) with a delay (Tm ).
The value initial of natal is
natal0(N)
= mnatal + 240 d(NP/4,N)/NP ,
where mnatal=5
And the value of ntl and natal through
the time will be
ntl t(N)
= (natal t(N) + 2 mnatal2)/(2
mnatal)
natal t+100(N)
= natal t(N) + (ntl
t(N) — natal t(N))/km
if
ec<1,
where km=Tm/100 and ec=E/E0.
Else
natal t+100(N) = natal
t(N)
2.3. Science System
The science system determines the probability of learning (PL)
for
each social behaviour in each subsystem. In the previous model, the probability
of learning is
PL(U,N) = P(U,N) + RE(N) åM¹NP(U,M)
EM(M) IMP(M,N)
where each individual system N has an emission
factor EM(N) and a reception factor RE(N).P(U,N)
is the probability of the behaviour U for the individual
system N.
We will express now the probability of learning with the formula
PL(U,N) = P(U,N) + RE(N) åM¹NP(U,M)
EM(M,U) IMP(M,N)
where EM(M,U) = åVP(V,M)
em(V,U) , and em(V,U) = f(U)V/15 expresses
the capacity of emision of learning of an individual system with behaviour
V on the behaviour U in other individual systems.
We use the REGINT(14) program to find the
better function f(U) (which have to decrease with U) in order to get a
dual evolution,
f(U) = 3.180501 + 0.004214 U2
– 0.210115 U
We can see in Table 1 the values of f(U) and the capacity of emision of learning on the same behaviour, for the most significative behaviours (Fº15º1111 and Eº14º1110 in hexadecimal, decimal and binary mode, respectively).
| U | 0 | 1 | 2 | 3 | 6 | 7 | E | F |
| f(U) | 3.180501 | 2.974600 | 2.777127 | 2.588082 | 2.071515 | 1.916182 | 1.064835 | 0.976926 |
| em(U,U) | 0 | 0.198306 | 0.370283 | 0.517616 | 0.828606 | 0.894218 | 0.993846 | 0.976926 |
2.4. Impact System
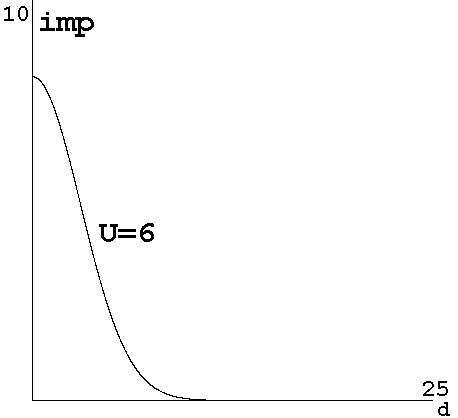
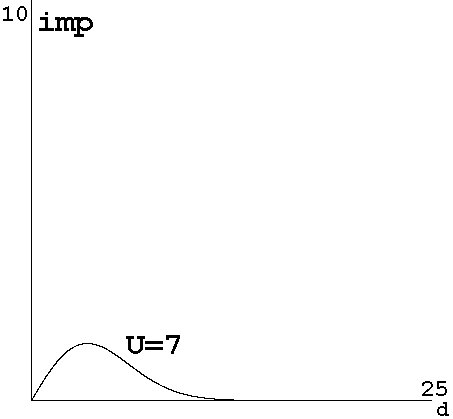
In the previous model, the impact (imp) decreased lineally with the distance, and it were maximum when d=0, that is to say, on the own system. This effect only affected to the repression (between different social behaviours).
Now, we will change the impact function so that
if U0
= 0 (gregarious behaviours), the impact is maximum when d=0,
decreases with d and increases with natal, but
if U0
= 1 (individualist behaviours), the impact is equal to zero
when d=0, that is to say, the repression which is
produced by such behaviours only acts on other social systems.
In the case of NP = 50 we will take:
If U<=3 then imp(U,M,N) = (natal(N)/0.4429U)1-U0exp
(-0.5c2) ((10.8+U)c)U0
If 4<=U<=7 then imp(U,M,N) =
(natal(N)/1.33859U)1-U0
exp (-0.5c2) (c(U+7.1)/6)U0
If 8<=U<=F then imp(U,M,N) = (natal(N)/0.8U)1-U0
exp (-0.5c2) (cU/90)U0
where c = d/dis, with dis = U/2 .
We can see in Figure 1 two examples of these
impact functions.
 |
 |
| Gregarious Impact | Individualist Impact |
Also, probability of recovery will be
a(U) = 0.09(1-2U/MM)
from a social behaviour U, where
j=0,1,…,mm-1, U=åjUj2jis
the binary numeric representation of the social behaviour U
º
(Umax-1,…,U1,U0),
MM=2mm –1 is
the maximum state-value 1…11 with maximum dimension mm, and 0.09 is a value
found in other works to avoid an early extinction of the population. Thus,
A(N) = 0.09 – 0.18 P(U,N) U / MM
for the probability of substitution of a destroyed individual subsystem
N.
Also, we will take
rej(Uj)
= Uj 2j
/MM
and therefore the capacity of reception of the behaviour U will be
re(U) = U/MM
On the other hand, might will be
m(U) = åjmj(Uj)
= åj Uj2j/(2
mm-1-1)
= U/7
and ferocity will be
n(U)
= 1-Õ
j [1- nj(Uj)
] = 1-Õ
j [1- (1-Uj)j/(mm-1) ] = 1-Õj/Uj=0
(3-j)/3
and therefore
st(U) = [2U/(MM-1)][1-Õj/Uj=0
(mm-1-j)/mm-1)]
We take Ktnt=20000, Kprg=10000 and NP=50, and we work with a maximum dimension mm=4 (and therefore MM=15) .
We speak about predominance of a state U if its probability is the majority (P>0.5) in a relative majority of subsystems. And we speak about strong predominance if moreover its probability of satisfaction (PG) is the maximum.
The Figure 2 shows graphically the relationships
of our Model of Dual Evolution of the Humanity.
Figure 2
Relationships in the Model
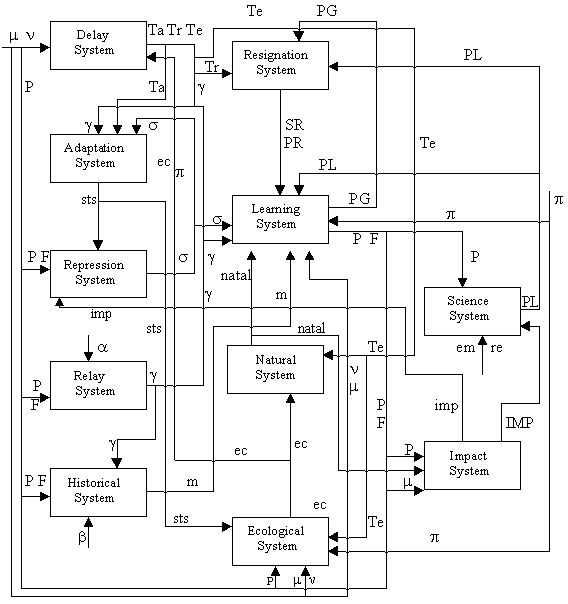
Mathematical relations in the model of Adaptive, Historical, Geographical and Multidimensional Evolution with Resignation
Resolution Level
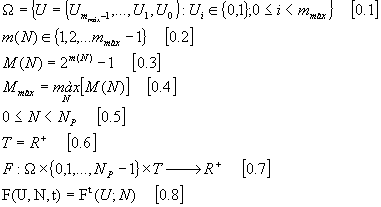
Learning System
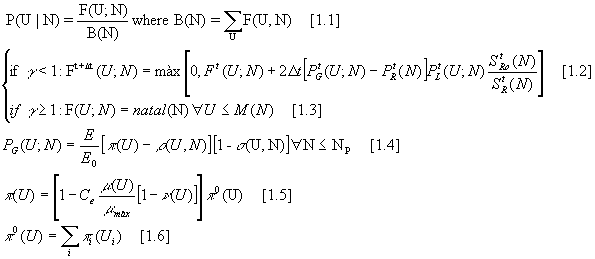
Repression System
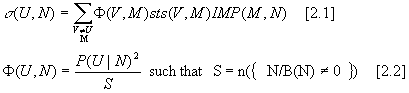
Science System
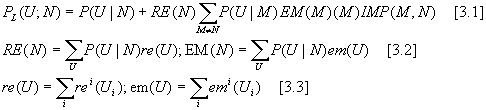
Relay system
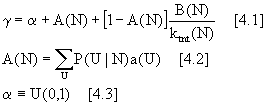
Historical System
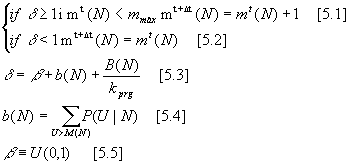
Adaptation System
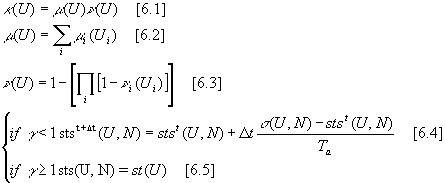
Resignation System
![]()
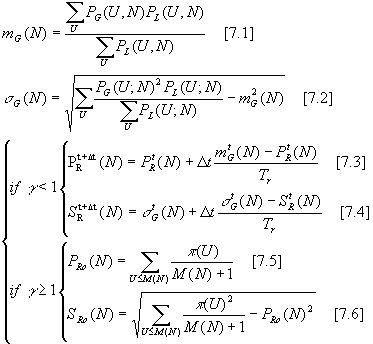
Ecological System
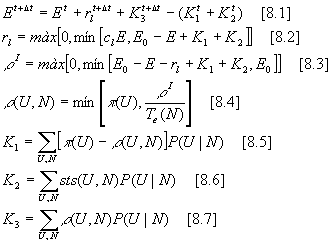
Delay system
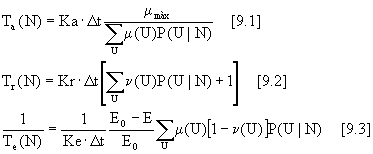
Impact System
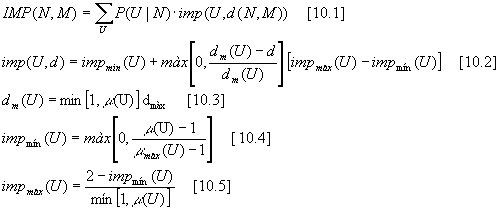
Natural System
![]()
A possible interpretation of the dimensions and behaviours would be
Dimension m=0: primitive society
Dimension m=1: agricultural revolution
U=0000=0:
Oriental Empire
U=0001=1:
Occidental Slavery
Dimension m=2:technological increase
U=0010=2:
Oriental Feudalism
U=0011=3:
Occidental Feudalism
Dimension m=3: industrial revolution
U=0110=6:
State Socialism
U=0111=7:
Capitalism
Dimension m=4: technological revolution
U=1110=E,
U=1111F: Free Scientific Society
Other values of U can represent anomalous social
behaviours (Fascism, Stalinism…) that appear for what we have called "revolt
effect"(9).
Unfortunately, the program stop without showing if a free scientific society would arrive to predominate…
If this result simulates the evolution of the humanity in the Earth planet, it will describe the capitalism as an unique mode of production in the world, without rivals neither alternative at short term. The survival of "state socialist societies" as China, North Korea, Vietnam or Cuba, would express a voluntarist desire of resisting to its widespread crumbling and, if we are abided by the course of the events, sooner or later they would finish being absorbed by the world capitalism(13).
This situation doesn't imply that the capitalism had triumphed forever
(our model not says so much), neither that it were the best social system:
on the contrary, in these system there are many social inequality, poverty.and
opression (specially between different countries), which only could be
overcome in the hardly reachable Free Scientific Society...
Figure 2
An example of dual evolution from the model
T=Time, EC=Ecology, S=Number of active systems
T Majority Behaviour in the Population (N from 1 to NP) EC S 0 00000000000000000000000000000000000000000000000000 1.00 31 100 --0000000000 000000-00000000000000000000-0-000000- 1.00 49 200 -1-00000000 -0000010-0000000000000000001010000001 1.00 48 300 1110000000 - 0000101000000000000000-001010000001 1.00 46 400 111000000 - 1 0001010-00-0 00-00-00-001-1-000001 1.00 44 500 11100000 1 1- 0-01010010 00100100-001111---001 1.00 42 600 1110000- 1 11 - 10-010010 0010-10--0011-1111001 1.00 42 700 1-1000 - -1 11 1 10101001- 0010110---01111111001 1.00 42 800 11-- - 1 11 11- - 1 1-10011 001011----011111-1--1 1.00 41 900 1111 1 1 1- 111 1 1 1110011 0010111---01111111111 1.00 41 1000 1111 1-1 1- -11 1 1 111001--001011----01-11111111 1.00 43 1100 1111 111 -1 111 1 1 111 01110010111---0-111111111 1.00 42 1200 1111 111 11 111-1 1 111 111001011--2-0-111111111 1.00 42 1300 1111 1-1 11- -1111-1 1-1 111001011--220--11111111 1.00 44 1400 1111-111 111 1-11111 111 11100101---22 --11111111 1.00 44 1500 11--11-1 111 1-11111 111 11100101---22 --11111111 1.00 44 1600 11111111 111 1311111 111 111 1-1---22 --11111111 1.00 42 1700 11111111-111 -311111-11- 11- 111--222 --11111111 1.00 44 1800 11111111-11- 33311111111- 111 111--222 22---11111 1.00 45 1900 -1-11-111113 333--1111111 111 11---222 2---1111-1 1.00 45 2000 -111111111-3 333--111111- 11- 11--2222 2-2-111--1 1.00 45 2100 1111111-11-3 333---111111 111 11-22222 2---111111 1.00 45 2200 111111113-33 333--1111111-11- 1-222222 2---111111 1.00 46 2300 111111113333 -3333-1-11111111 --222222 2-----1111 1.00 46 2400 11--111-33-3 -3-33-11-1111111- --222222-2-----111- 1.00 48 2500 1111113--3-3 -3-33-311111111-- 22-22222-2------1-1 1.00 48 2600 111111-3-3-3 -3--333-11111111- 22-222--22------111 1.00 48 2700 111-1--3-3-3 -3---33-11-1111----2-222--2--------11 1.00 49 2800 --1------3-3 -3-3-33--11-11-----2--22--2---------1 1.00 49 2900 ---------3-- -3----33-11111-----27--2----7-------- 1.00 49 3000 ----------7- 737----331-111-----2 --2-6--6-------- 1.00 48 3100 --------7-77 737---------11------ ----6----------- 1.00 48 3200 --------7-77 7-7---------11------ ----6----------- 1.00 48 3300 --------7777 7-7-7-7------------- ----66---------- 1.00 48 3400 -----77-7777 7-7-777------------- ---66666-------- 0.97 48 3500 -----77--777-7-777777------------ -66666666------- 0.91 49 3600 ----777--77-777777777------------ 6666-6666666---- 0.81 49 3700 ---777-77777777777777----------6- 6666-6666666---- 0.68 49 3800 ---777-777777777-7777-7--------6- 6666--666666---- 0.50 49 3900 --7777 -777 7777-7777 77-------6- 66-6--666 66---- 0.33 45 4000 --5777 77 7 7 7777 7 - 5 --6 6--6-A-6 66E - 0.21 33 4005 -- 777 77 7 - 7777 7 - --- ---6- -6 66 - 0.16 28 4105 - 777 7 7 7777 7 - 6-- -- 6- -6 6- - 0.21 24 4205 - 7 - 7 7 7-77 - 6- - 6 - 6- - 0.28 18 4305 - - 5 7 7 7-77 - 6- 6 6 - 0.41 15 4310 - - 7 7 7-77 - 6- 6 6 - 0.62 14 4410 - - 7 7 7 77 - 6- 6 - F 0.92 13 4510 - 7 7 7 77 - 6 6 - F 1.00 11 4610 - -7 7 7 77 - - 6 6 - - 1.00 13 4710 - -7 7 7 77 - 7 - - 6 - - 1.00 14 4810 - 77 7 7 77 7 7 - - 6 - - 1.00 14 4910 - 77 7 7 7- - 7 - - 6 - - 1.00 14 5010 - 77 7 -7 7- - 7 - - - - - 1.00 15 5110 -- 7- 7 -7 77 7 7 - - - - - - 1.00 17 5210 -- 7- 7 -77 77 7 -7 - - - - - - 1.00 19 5310 -- 77 - 777 77 7 -7 - - - - - - 1.00 19 5410 -- - 77 7 77- -7 7 -7 - - - - - - 1.00 20 5510 -- - -7 7 777 77 7 77 - - - - - - 1.00 20 5610 -- 7 77 7 777 77 7 77 - - - - - - 1.00 20 5710 -- 7 77 7 777 77 7 77 7 - - - - - 1.00 20 5810 77 7 77 7 777 77 7 7- 7 - - - - - 1.00 20 5910 77 7 77 7 777 77 7 7- 7 - - - -- 7 1.00 21 6010 7- 7 77 7 777 77 7 77 7 - - - 7- 7 1.00 21 6110 7- 7 77 7 777 77 7 77 7 - - - 7- 7 1.00 21 6210 77 7 77 7 7-- 77 7 77 7 7 - - 7- 7 1.00 21 6310 77 7 77 7 777 77 7 77 7 7 7 7- 77 7 1.00 22 6410 77 7 77 7 777 77 7 77 7 7 7 7- 77 7 1.00 22 6510 77 7 77 7 777 77 7 77 7 7 7 7- 77 7 1.00 22 6610 77 7 77 7 777 77 - 77 7 7 7 7- 77 7 1.00 22 6710 77 7 77 - 777 77 - 77 7 7 7 7- 77 7 1.00 22 6810 77 7 77 7 7-7 -7 7 -7 7 7 7 77 77 7 1.00 22 6910 -7 7 77 7 777 77 7 -7 7 7 7 77 77 7 1.00 22 7010 -7 7 77 7 777 77 7 -7 7 7 7 77 7- 7 1.00 22 7110 77 - 77 7 777 77 7 77 7 7 7 77 -- 7 1.00 22 7210 7- 7 -- 7 777 77 7 77 - 7 7 -- -7 7 1.00 22 7310 7- 7 77 7 777 77 7 77 - 7 7 -- 77 7 1.00 22 7410 77 -7 77 7 777 77 7 77 7 7 7 -- 77 - 1.00 23 7510 77 -7 77 - 777 77 7 77 7 7 - 7- 77 - 1.00 23 7610 -7 77 77 7 777 77 7 77 7 7 - 7- 77 7 1.00 23 7710 -7 77 77 7 777 77 - 77 7 7 - 7- 77 7 1.00 23 7810 77 77 77 - -7- 77 - 77 7 7 7 77 77 7 1.00 23 7910 7- 7- 77 7 777 77 7 77 - 7 7 77 77 7 1.00 23 8010 -7 77 77 7 777 77 7 77 - 7 7 77 7- 7 1.00 23 8110 -7 77 77 7 7-7 77 7 77 7 7 7- 7- 7- 7 1.00 24 8210 77 77 77 7 777 7- 7 77 7 7 7- 7- 77 7 1.00 24 8310 77 77 77 7 77- -7 7 77 7 7 7- -- 77 7 1.00 24 8410 77 77 77 - 777 77 7 77 7 7 77 -- 77 7 1.00 24 8510 77 77 7- 7 777 77 7 77 7 7 77 7- 77 7 1.00 24 8610 77 77 77 7 777 77 7 7- - 7 77 7- 77 7 1.00 24 8710 77 -7 77 7 777 77 7 7- - 7 77 77 77 7 1.00 24 8810 77 77 77 7 777 77 - 77 7 7 77 77 77 7 1.00 24 8910 77 77 77 7 777 77 - 77 7 7 77 77 -7 7 1.00 24 9010 77 77 77 7 777 77 7 77 7 7 77 77 77 7 1.00 24 9110 7- 77 -7 - 777 77 7 77 7 7 77 7- 77 7 1.00 24 9210 77 77 77 7 777 77 7 -7 7 7 77 7- 7- 7 1.00 24 9310 77 7- 77 7 -77 77 7 -7 7 7 77 7- 7- 7 1.00 24 9410 77 77 77 7 77- 77 7 -7 7 7 77 77 77 - 1.00 24 9510 77 77 77 7 777 77 7 77 7 7 77 77 77 7 1.00 24 9610 -7 77 77 7 777 77 7 77 7 - 77 77 77 7 1.00 24 9710 77 77 77 7 777 -7 7 77 7 - 77 77 -7 7 1.00 24 9810 77 77 77 7 777 77 7 7- 7 7 -7 77 -7 7 1.00 24 9910 77 77 77 7 777 77 7 7- 7 7 -7 77 77 7 1.00 24 10010 77 7- -77 7 777 77 7 77 7 7 77 77 77 - 1.00 25
REFERENCES